02/19/2021
Simulation of Transient heat transfer in eggs
Teammates
Aaron Juchau, Matt Shulman
All three of us simulated the process in COMSOL separately before coming together to analyze the data.
GOAl
The goal of this COMSOL Multiphysics simulation was to determine the proper time necessary to cook a soft boiled egg. The numerical solution to this transient scenario will then be compared to experimental collected data.
Introduction
The provided dimensions for our egg are 4.2 cm in width and 5.6 cm in length. According to the research paper “Numerical modeling of heat transfer and pasteurizing value during thermal processing of intact egg” an empirically accurate ratio of volume of egg white to egg yolk is 26.5% egg yolk, therefore, we chose to proceed with this value as our guide for our simulation over the provided 33%. In all of our simulations our egg yolk radius ranged from 1.25 - 1.48 cm.
This simulation is based on the heat conduction equation, where c is specific heat, ⍴ is density and K is thermal conductivity. These values are what were input into COMSOL in order to define the heat conduction of the egg.
In order to determine the correct amount of “doneness” for the soft boiled egg, we used the range 144-149 degrees Fahrenheit as our guide for ensuring the egg was cooked to a soft boiled state. The heat transfer in boiling an egg is convection, governed by the following equation.
There are numerous assumptions that had to be made in order to complete the simulation on COMSOL.
Assumptions
The egg yolk and white are modeled as solids
Internal convection and associated momentum balances are neglected
The egg’s shell is a regular ellipsoid with elliptical cross-sections
The egg’s yolk is a sphere with circular cross-sections
The properties of specific heat, density, and conduction coefficient for the egg yolk and white are independent of time and temperature
The heat transfer rate from the boiling water is constant
The water is at a constant temperature of 100°C (373 K)
The pot is continuously heated, and the heat capacity of the pot and water is much greater than that of the egg
Heat transfer from the water to the egg is via convection1
The volume of the egg is entirely filled with yolk and white; there is no air space
The location of the air space would depend on the orientation of the egg in the pot
The boundaries between the yolk, white, shell, and water are otherwise impermeable
No mass transfer across boundaries
The egg’s material properties and geometry are axisymmetric about the major axis of the ellipsoid
All heat transfer inside the egg is conductive, ie. there is no convection within the egg
Process
In order to fully understand the heat transfer that occurs in egg boiling, we modeled the egg with and without a shell in order to determine if the shell changed the rate at which the egg cooked. In both of our models we chose to model the egg as axisymmetric about the center of the ellipse. The yolk was modeled using COMSOL’s built in subtraction feature in order to define distinct regions of the egg. The same was done for the model that included the shell. The below image shows the location we chose to select probes in the COMSOL model. These probes would allow us to see the distinct temperature over a range of time at each of these locations. The egg white and yolk were given the initial temperature of 68 degrees Fahrenheit. Collecting data every 3o seconds could allow us to determine the correct temperature and time for the egg to be cooked to soft boiled.
RESULTS
The below chart shows the temperature distribution along the major axis of the modeled egg with the shell at 11 different times, spaced at 30 second intervals. From this data we determined that the egg would reach the cooked value of 146 degrees Fahrenheit at approximately 240 seconds, while keeping the yolk runny. When comparing this to the version without the shell, it showed very minimal difference in the rate of heat transfer from the boiling water to the egg.
The following is a visual representation of the heat transfer that changes over time. In analyzing both this visual and the numerical data we determined that there would be a bit of inconsistency due to the elliptical shape of the egg. The shape will result in a tiny portion of the egg yolk to be cooked and the white uncooked. Using the heat transfer plots we were able to decide that 270 seconds is just the right amount of time to get a runny egg yolk and cooked white, making the perfect soft boiled egg.





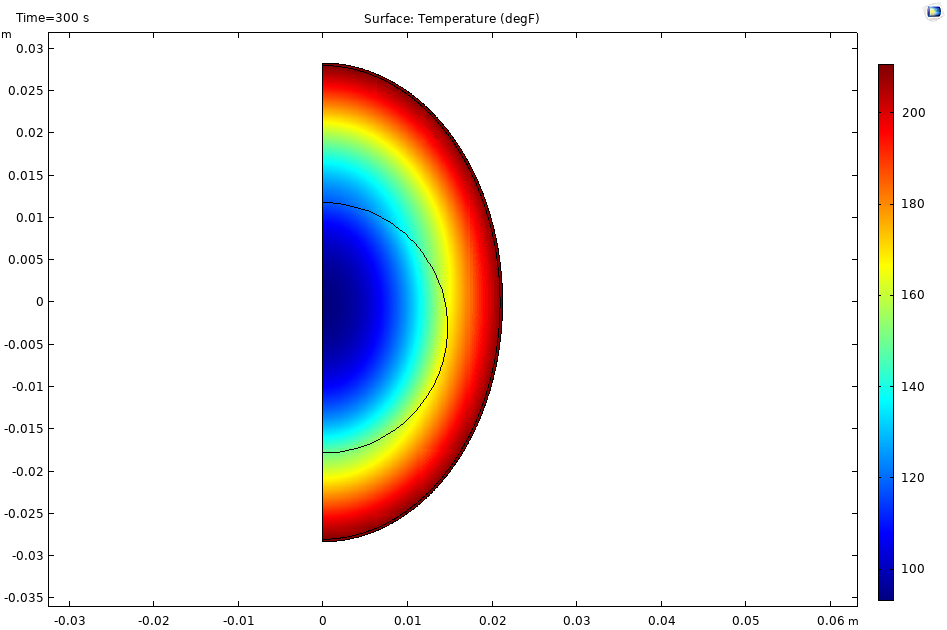
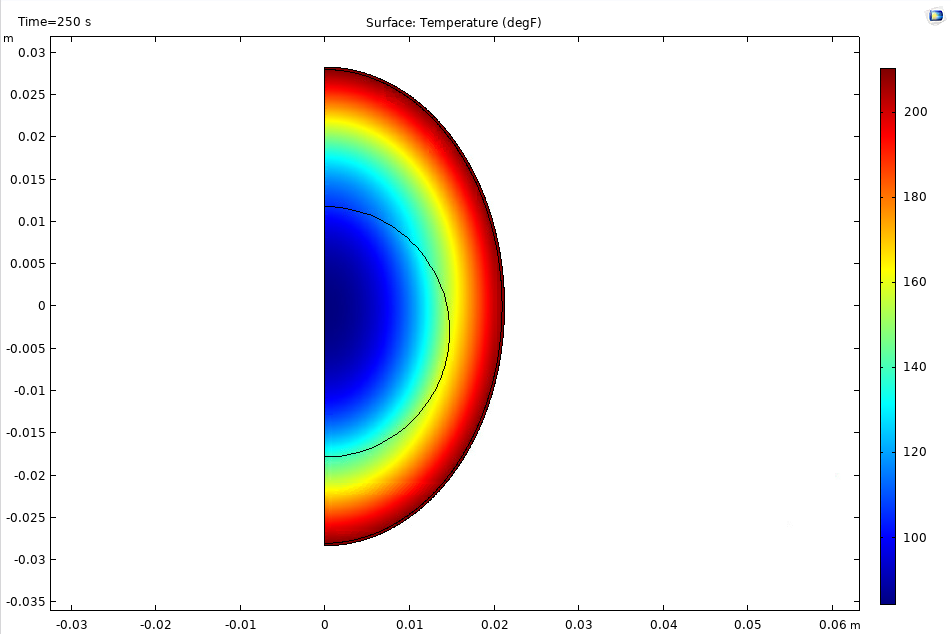
The following shows how minimal the difference between the simulation with and without the shell are. While the color variation is clear in the left image (without the shell), if you zoom into the color distribution of the right image (with the shell), it is actually very similar but on a smaller scale. The difference is really only clear at t=0 and it begins to even out as time passes.
Experimental Data
In order to determine if 270 seconds was the correct amount of time and analyze the validity of the simulation we boiled a hard boiled egg beginning at room temperature. You can see that there is a bit of cooked yolk and runny white, consistent with our predictions based on the size and shape of the egg. Cooking eggs at 240 and 300 seconds further solidified the results of our COMSOL simulation.







